Ranked Choice Voting
Vote Your Hopes, Not Your Fears

Voting Goals

1. Elect representatives who understand and represent the needs of the greatest possible number of citizens.
2. Help people feel comfortable voting for the candidate who best represents their hopes.
3. Encourage positive campaigns based on factual, relevant information.
4. Reduce costs for governments and candidates.

Why Change?

Do you want to keep getting what you always got? If your answer is "yes", thanks for stopping by. If not, please keep reading.

It should not matter how many candidates are running or how votes split among like-minded candidates. With only 2 candidates, except in rare cases of a tie, one candidate wins with majority support which meets goal #1. What happens if there are more than two candidates? Sometimes a candidate opposed by most voters wins. Could two candidates be considered the "real" contenders with independent or other-party candidates considered "vote-stealing spoilers"? Could voters feel like a vote for one of the "other" candidates is wasted, or fear that the winner could be the candidate they want least?

It has been said a big part of life is just showing up. The voters who show up for a primary election determine the options for everyone showing up for the general election. Governments and candidates pay for all elections regardless of how many show up to vote. If there were several candidates in the general election and a runoff is needed, the two runoff candidates could be polar opposites with no middle ground. How might voters feel about their voting choices? Is it possible that many voters might prefer a candidate who did not make it into the general election or a runoff?

In multi-seat, at-large elections such as city councils, school boards, or county supervisors, should the largest voting bloc choose all the winners even if that group represents less than half the voters?

Do negative campaigns or attack ads give voters the information they want and need to choose an effective representative?

What is a "spoiler" candidate?

An independent or other-party candidate with little voter support may share many values of another candidate considered "electable" or a "serious-contender". Each vote for this "other" candidate is one less vote for the "electable" candidates and may throw the election to the least desirable candidate.

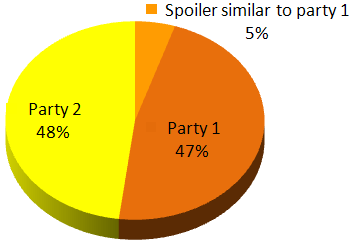
In the above example, the "spoiler" shares much in common with Party 1. If the spoiler wasn’t in the race, most of the spoiler’s supporters would vote for the Party 1 candidate who would then win with over 50% of the vote. As it is, the candidate from Party 2 wins with 48% of the vote.

How Can Ranked Choice Voting (RCV), also known as Instant Runoff Voting (IRV), Help Meet the Goals?
It is as Easy as 1-2-3

In many of our life decisions, we don’t just choose one option, and then let others choose for us if our top selection isn’t available. We prioritize items on our to-do lists. We make backup or contingency plans if our first effort doesn’t work out. If our first choice isn’t available, we expect the opportunity to make another selection from all the remaining options. If the ice cream store is out of our favorite flavor, we want to select an alternative from all the store’s flavors, not just the two flavors chosen most often by other customers. Why should we settle for less when we choose those who influence many aspects of our lives?

• The simplicity of a single election to determine the winner

• A familiar process regardless of the number of candidates

• The power to express your preference for every candidate

Ranked choice voting (RCV), also known as Instant-Runoff Voting (IRV) or Single-Transferable Vote (STV), fulfills the above goals. In an RCV election, voters rank candidates in order of preference, much like prioritizing items on a to-do list. Voters who want to keep it simple can mark just one candidate and leave the other selections blank. Those who have nuanced opinions can express their preference for every candidate.

Primary elections ask voters "who is your most preferred candidate?". General or runoff elections ask, "Who is your most-preferred (or least-hated) candidate of those remaining?". RCV elections ask both questions on the same ballot. RCV uses the familiar process of a runoff between a reduced number of candidates but gets results with one election because voters are asked for their 2nd (and 3rd, 4th, etc.) choices on one ballot. Imagine multiple runoff elections with one less name on each runoff ballot but you only need to cast one ballot. The vote-counting computer has the information it needs to tabulate as many runoffs as required until one candidate gets over half of the votes. See the example below.

RCV has the obvious result of eliminating the need (and cost) of a separate runoff election if there’s no majority winner in the first round.

RCV builds consensus as voters who have their first-choice candidate eliminated in one round can choose a like-minded candidate in the next runoff round. A voting bloc with three candidates running will reach consensus on one of the three through multiple runoff rounds of vote counts. This eliminates problems due to multiple, similar candidates splitting the vote and unintentionally contributing to a win by a very different candidate.

"Long-shot" candidates will not affect the outcome. If they really have few supporters, they will be eliminated in an early round and those who voted for them can cast their second-choice vote for a "mainstream" candidate. If the candidate has many sympathizers, it could lead to a surprise upset as voters can vote for anyone as their first choice without fear of unintended consequences.

RCV helps weed out extreme candidates as voters from a smaller voting bloc may influence which candidate from a majority party or voting bloc wins.

RCV discourages negative campaigning. Suppose you’re ranking candidates A, B, and C. The race is close, and the winner will need some second-choice votes to reach the 50% threshold. You fully support candidate B as your first choice, but are uncertain how to rank A or C. Then candidate A says, "Candidate B eats puppies. Don’t vote for that puppy killer". Will that influence who gets your second-choice vote?

RCV also works for multi-winner elections such as city councils, school boards, and county supervisors, and achieves the goal of giving each voting bloc a fair share of power.

The links page has more on RCV and where it is used now.

To help visualize the process, imagine that all voters and all candidates gather in one place.

1. Everyone lines up behind their favorite candidate.
2. Count the people in each line. If over half the voters are in one line, that candidate wins.
3. If there’s no winner, one candidate with the shortest line loses. Each voter in that line either moves to a different line for an immediate runoff round or goes home. Go back to step 2 and count everyone again.

You do not have to rank all the candidates but only ranking one or a few is like going home before the election is over. Each voter gets one vote in every round and all votes count equally. The vote-counting computer follows a procedure like the visualization above.

• Goal #1 : Over half the voters support the winner and non-majority voters can have a say by casting a second-choice vote for their least objectionable majority-party candidate.
• Goal #2 : People vote their hopes, not their fears which leads to more enthusiastic voter participation. Since voters get second and third (or more) choices, votes for third-party, independent, or write-in candidates aren’t "wasted". Candidates with little support don’t become "spoilers" and hurt the chances of other candidates.
• Goal #3 : RCV can reduce negative campaigns and attack ads. Candidates may need second-choice votes to win which requires reaching out to all voters. Trashing a voter’s first choice candidate reduces the chance of getting that voter’s second-choice vote.
• Goal #4 : No more separate runoff elections. Governments and candidates save money.

Choose 1 winner from 3 candidates

Round 1

12 Votes

10 Votes

8 Votes

One of the goals is to build consensus. Nobody got a majority, so we continue to round 2. The purple candidate got the fewest votes and is eliminated. The computer now counts everyone’s votes again, this time using the second-choice votes of everyone who selected purple as their first choice. 6 of them selected orange as their second choice, 2 selected gray.

Round 2

14 Votes
 

16 Votes
The Winner

Eliminated
 

If there are more than three candidates, the process would repeat as needed. One candidate is eliminated at the end of each runoff round, and all votes are counted again in each following runoff round until one candidate gets over half the votes.

Voting for Just One is So Much Easier

You can still vote for just your first choice if you wish. Those who prefer to express their thoughts about several or all of the candidates have that additional power.

Having just two options is simple and convenient in some ways. If you hear something you like about one, vote for that one. If you hear something you do not like, vote for the other one. This simplicity comes at a very high price if you do not like either option, or if the options are polar opposites and you want something in the middle.

The first time I voted using ranked choice voting, it seemed daunting. I only knew some of the candidates. As I learned about all the candidates, I realized I had preferences. I decided on a "divide and conquer" approach. I first divided the candidates into three groups: most preferred, acceptable, and less preferred. You may choose different groups, or a different number of groups. Now there were only a few candidates in each group, and it was easy to assign rankings within the group.

Giving More Voters a Voice in Single-Winner Elections

A tabulating procedure known as Condorcet uses the same ranked ballots but tabulates them differently. Rather than calculate a series of runoff rounds with one candidate eliminated each round, Condorcet calculates a series of one-on-one elections pitting each candidate against every other candidate, one at a time. The winner is the candidate who beats all others. In each round, the computer checks each ballot for the two candidates in the current contest and chooses the highest-ranked candidate of the two as that voter’s choice. See the sample election below. The two candidates considered in a round might be the first and second choices on one voter’s ballot, and the third and fifth choices on another voter’s ballot.

Advantages of Condorcet

Condorcet gives voters in a smaller voting bloc a greater say in which candidate from the majority party or voting bloc wins. This can lead to winners closer to the center which could help depolarize districts where an extreme majority party or voting bloc has a lock on the outcome and smaller voting blocs are marginalized.

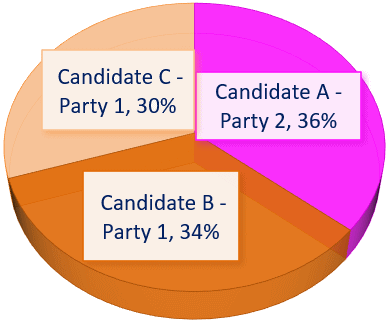
The above diagram shows a very close race with Party 1 candidates B and C combined having a 64% majority so we would expect a winner from Party 1.

Using a traditional runoff between candidates A and B denies Party 2 voters the opportunity to express a preference for candidate C over candidate B.

Using RCV rules, Candidate C of Party 1 would be eliminated as this candidate has the fewest votes. Assuming those who selected Candidate C as their first choice remain loyal to their party, most of their second-choice votes would go to Candidate B of Party 1 making Candidate B the winner. Even though Candidate B wins and will represent all voters, Party 2 voters had no say in which candidate of Party 1 represents them. Party 2 voters could select a Party 1 candidate as their first choice, but that violates Goal #2. It is also possible that many Party 1 voters are upset with both Party 1 candidates and might prefer the Party 2 candidate. The voting system should be flexible enough to support all possibilities.

Using Condorcet vote tallying, and assuming most Party 1 voters remain loyal to their party, both Party 1 candidates would beat the Party 2 candidate. The contest between the two Party 1 candidates now depends on the second-choice votes of Party 2 voters. Party 2 voters would likely choose the Party 1 candidate who is closer to the center. If most Party 2 voters prefer Party 1 Candidate C then Candidate C would win and represent all voters in both parties.

Disadvantages of Condorcet

• Condorcet may produce a winner who got few first-choice votes but lots of second-choice and third-choice votes. A winning strategy might be to avoid offending anyone by never taking a strong stand on anything. In the absence of strong polarization or extreme candidates, it might be better to have a winner who has the support of many voters rather than a winner who is least objectionable to most. Keep reading for an alternative using RCV, multi-member districts, and proportional representation.
• Condorcet does not guarantee a winner and needs a fallback plan such as RCV if there is no Condorcet winner.
  • Candidate A could beat candidate B but lose to candidate C
  • Candidate B could beat candidate C but lose to candidate A
  • Candidate C could beat candidate A but lose to candidate B

Ranked Voting Paves the Way to Proportional Representation

If you are not at the table, you are on the menu.

Consider a one-round election for school board with the candidates who get the most votes win. If there are three voting blocs, one with 40% of the voters and two groups each with 30% of the voters, the entire school board could be chosen by 40% of the voters. If five candidates are elected by district, each district contains about 20% of the county’s voters. A majority in each district is just over 10% of all voters in the city, county, or district. 11% of the voters could elect a representative objectionable to the other 89%.

In the recent past, court decisions required that minority groups have some representation on a five-member school board. In the above diagram, the green group is 68% of the population and the yellow group is 32%. How would you draw five districts, each with equal numbers of voters, to fairly represent everyone? If you drew districts around the two largest yellow areas, that leaves voters in the smaller yellow areas cut off with little or no influence.

Proportional representation provides majority control with minority representation. In this context, "minority representation" refers to any voting bloc consisting of less than half the voters. A voting bloc may be based on party, philosophy, economic interests, race, ethnicity, or anything else that is important to many voters in the current election. Voting blocs may shift but proportional representation automatically keeps up. Why have minority representation?

• If smaller voting blocs have a seat at the table and can influence decisions, they are more likely to be allies than adversaries.
• To have a government that understands the needs of most citizens.
• There may be costs or other consequences of continually brushing aside concerns of smaller groups.
• There may come a day when you are in the minority on some issue.
• To help meet Goal #1.
• To help guard against a complacent majority making poorly informed decisions with unintended consequences.

A majority group of voters elects at least three members of a 5-member governing body, but smaller voting blocs also get some representation by electing the remaining members. The five representatives together represent nearly all voters and discussions among representatives are likely to mirror discussions in the community.

Who gets a seat at the table, and how many seats should a voting bloc get?

If the largest voting bloc includes 3/5ths of the voters, and 2 smaller voting blocs each include 1/5th of the voters, we would expect the largest group to get 3 of 5 council seats for majority control, and the other 2 groups to each get one seat for minority representation. Power is fairly allocated among the various groups. It is important to understand that the groups are fluid and divisions may change from one election to the next. One election, the groups might be based on ethnicity. The next election, the divisions may be philosophical differences about a community issue that spans ethnic lines.

If there are more voting blocs than seats, only the larger groups get a seat, but everyone has a say about all the candidates. Smaller groups might build consensus by ranking candidates in a similar way and together select one winning candidate.

How does it work?

Imagine all voters and candidates gathering in one place to elect five representatives.

1. Everyone finds their favorite candidate and starts to form lines.
2. Any line with just over 1/6 of the voters is an instant winner and that line is closed. Voters who were heading for that now-closed line may choose a different line, knowing that their favorite candidate is already elected, or they may go home. If there are five candidates each with over 1/6 of the voters in their line, over 5/6 of the voters are in line behind winners and the election is over.
3. Until there are five winners, one candidate with the shortest line loses. Each voter in that line either moves to a different line or goes home. Go back to step 2 and count everyone again.

This achieves the goal of minority representation since any voter group including over 1/6 of the voters elects at least one representative, and all representatives together represent over 5/6 of the voters. All the losers together got less than 1/6 of the total vote.

Another goal is majority control. If over half the voters were heading for a winning line, we expect like-minded candidates to have a majority on the council. The winning candidate keeps just enough votes to win, freeing the rest of the voters to move to the line of another like-minded candidate which results in the desired majority control.

Multi-winner RCV builds consensus, allocates power fairly, and gives everyone a say about all the winners. It also makes gerrymandering irrelevant as power is automatically distributed among voting blocs, not according to district boundaries. Multi-winner RCV could be used to elect United States congress members by switching from single-member congressional districts to multi-member districts. Changing the number of district members requires congress to change to federal law.

Casting ballots is like other ranked voting elections: Rank the candidates in order of preference. The vote-counting computer really earns its electrons with this one.

You may skip this next section if you wish. The computer does all the calculations.

Determining winners requires some 5th-grade math such as fractions, percentages, and decimal places.

Why set the minimum votes to win at 1/6 rather than 1/5 for 5 seats? If one candidate gets over 1/5 of the votes, there are less than 4/5s left, not enough to elect 4 more winners. If the threshold is less than 1/6, there may be enough votes to elect more than 5 winners. When the 5 winners collectively get over 5/6 of the votes, there aren’t enough votes left to elect another winner.

The number of desired winners is not always 5. The computer calculates the minimum votes to win using the formula (1/(NumberOfWinners + 1))+1; If there should be three winners, the minimum is 1/4 of the total votes plus one more vote. If there is only one winner, the minimum is 1/2 of the total votes plus one more vote.

If a candidate receives more than the minimum number of votes needed to win, the computer uses fractions and decimal places to ensure that everyone who voted for the winner will be considered equally in later rounds.

Hand counting is possible using simpler methods.

Choose 3 winners from 5 candidates
The Orange Clique vs. the Purple Clique

50 voters total: 30 purple, 20 orange 13 votes are needed to win (1/4 of 50 + 1).

Fair representation suggests two purple winners and one orange winner to give majority control to the purple clique (30 of 50 votes or 60%), with minority representation for the orange clique (20 of 50 votes or 40%).

Round 1

11 Votes
 

9 Votes
 

16 Votes
Winner

8 Votes
 

6 Votes
 

The purple/yellow candidate got 16 votes which is enough to be elected, with 3 votes to spare. If we stopped here, the winners would be the purple/yellow, orange/gray, and orange/purple candidates which violates the goal of fair representation as the minority orange clique would have 2 representatives and the majority purple clique only one. We’ll keep going to find the next 2 winners. The 3 surplus purple/yellow votes now transfer to another candidate based on each voter’s second choice. 10 of the 16 purple/yellow voters selected the purple/orange candidate as their second choice. The other 6 purple/yellow voters selected the purple/gray candidate as their second choice.

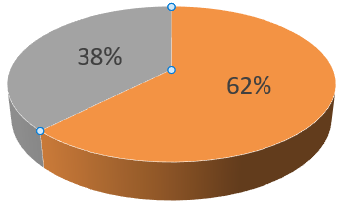
The computer can calculate this vote split precisely as (62.5% of 3) votes for the purple/orange candidate, and (37.5% of 3) votes for the purple/gray candidate. We’ll approximate that in the example below as 2 more votes for the purple/orange candidate and 1 more vote for the purple/gray candidate. Note that the total number of votes stays the same, and each voter has exactly 1 vote.

Round 2

11 Votes
 

9 Votes
 

13 Votes
Winner

10 Votes
 

7 Votes
 

Still no second winner as no other candidate got more than the required 13 votes. The computer follows the next RCV rule and eliminates the candidate with the fewest votes. In this example, the purple/gray candidate is eliminated, and the computer looks at the second-choice votes of all the purple/gray voters. All the purple/gray voters selected the purple/orange candidate as their next choice which makes purple/orange the next winner.

Round 3

11 Votes
 

9 Votes
 

13 Votes
Winner

17 Votes
Winner

Eliminated

The computer continues following the RCV rules to find the third winner, one of the orange candidates.

Proportional Representation Makes Gerrymandering Irrelevant

Is Ranked Choice Voting for Real?